5 Steps To Master Absolute Value Equations

Quick Links :

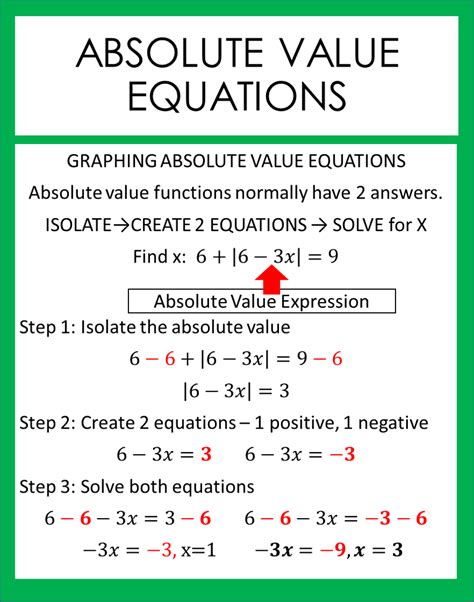
Mastering absolute value equations is a crucial skill for any student of mathematics. Absolute value equations are used to describe situations where the distance from a certain point is of interest, rather than the direction. In this article, we will explore the concept of absolute value equations and provide a step-by-step guide on how to solve them.

Absolute value equations are equations that involve the absolute value function, denoted by |x|. The absolute value function is defined as the distance from zero on the number line, without considering direction. For example, |3| and |-3| both equal 3, since they are both 3 units away from zero.

Here's an example of an absolute value equation: |x| = 5. This equation can be read as "the distance from x to zero is 5." To solve this equation, we need to find the values of x that satisfy this condition.

In this article, we will outline the 5 steps to master absolute value equations.

Step 1: Understand the Definition of Absolute Value

Before diving into solving absolute value equations, it's essential to understand the definition of absolute value. As mentioned earlier, absolute value is the distance from zero on the number line, without considering direction. This means that absolute value is always non-negative, since it represents a distance.

For example, |x| = |y| means that the distance from x to zero is the same as the distance from y to zero. This does not necessarily mean that x equals y, since x and y can be on opposite sides of zero.

Understanding Absolute Value

Step 2: Write the Equation in Standard Form

To solve an absolute value equation, it's crucial to write the equation in standard form. The standard form of an absolute value equation is |x| = a, where a is a constant. If the equation is not in standard form, rewrite it to match this format.

For example, |2x - 3| = 5 is in standard form, while 2|x - 3| = 5 is not. To rewrite the latter equation, distribute the 2 inside the absolute value: |x - 3| = 5/2.

Writing the Equation in Standard Form

Step 3: Set Up Two Equations

Once the equation is in standard form, set up two equations to solve. The first equation sets the expression inside the absolute value equal to the constant: x = a. The second equation sets the expression inside the absolute value equal to the negative of the constant: x = -a.

For example, if the equation is |x| = 5, set up the following two equations: x = 5 and x = -5.

Setting Up Two Equations

Step 4: Solve Each Equation

Solve each of the two equations set up in Step 3. If the equation is simple, solve it by adding, subtracting, multiplying, or dividing both sides by the same value.

For example, solve the equations x = 5 and x = -5. The solutions are x = 5 and x = -5, respectively.

Solving Each Equation

Step 5: Write the Final Solution

After solving each equation, write the final solution. The final solution includes all the solutions obtained in Step 4.

For example, if the solutions to the equations x = 5 and x = -5 are x = 5 and x = -5, respectively, the final solution is x = 5 or x = -5.

Writing the Final Solution

In conclusion, solving absolute value equations requires understanding the definition of absolute value, writing the equation in standard form, setting up two equations, solving each equation, and writing the final solution. By following these steps, you can master absolute value equations and tackle more complex math problems.

What are your thoughts on absolute value equations? Share your experiences and tips for solving these types of equations in the comments below.

Gallery of Absolute Value Equations

FAQs