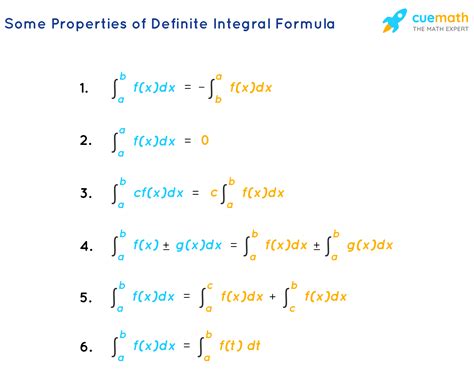
The quotient rule for integrals is a fundamental concept in calculus that can seem daunting at first, but with the right approach, it can be mastered in no time. In this article, we will break down the quotient rule into 5 easy steps, providing you with a comprehensive understanding of this powerful technique.
What is the Quotient Rule for Integrals?
The quotient rule for integrals is a method for integrating functions that can be expressed as the ratio of two functions. It is a powerful tool for solving complex integration problems and is widely used in mathematics, physics, and engineering. The quotient rule states that if we have a function of the form:
f(x) = g(x) / h(x)
where g(x) and h(x) are both functions of x, then the integral of f(x) can be evaluated using the following formula:
∫f(x) dx = ∫g(x) / h(x) dx
Step 1: Identify the Functions g(x) and h(x)
The first step in applying the quotient rule is to identify the functions g(x) and h(x). These functions should be clearly defined and should not be equal to zero. In most cases, g(x) will be the numerator of the ratio and h(x) will be the denominator.

Step 2: Evaluate the Derivative of h(x)
The second step is to evaluate the derivative of h(x). This is a critical step, as the derivative of h(x) will be used in the quotient rule formula. Make sure to use the correct rules of differentiation to evaluate the derivative of h(x).

Step 3: Evaluate the Integral of g(x)
The third step is to evaluate the integral of g(x). This can be done using a variety of techniques, including substitution, integration by parts, or integration by partial fractions.

Step 4: Apply the Quotient Rule Formula
The fourth step is to apply the quotient rule formula. This involves substituting the derivative of h(x) and the integral of g(x) into the quotient rule formula.

Step 5: Simplify the Result
The final step is to simplify the result. This may involve canceling out common factors, combining like terms, or evaluating any remaining integrals.

Example Problem
To illustrate the quotient rule, let's consider the following example problem:
Evaluate the integral:
∫(2x + 1) / (x^2 + 1) dx
Using the quotient rule, we can evaluate this integral as follows:
Step 1: Identify the functions g(x) and h(x)
g(x) = 2x + 1 h(x) = x^2 + 1
Step 2: Evaluate the derivative of h(x)
h'(x) = 2x
Step 3: Evaluate the integral of g(x)
∫g(x) dx = ∫(2x + 1) dx = x^2 + x + C
Step 4: Apply the quotient rule formula
∫f(x) dx = ∫g(x) / h(x) dx = ∫(2x + 1) / (x^2 + 1) dx = (x^2 + x + C) / (x^2 + 1)
Step 5: Simplify the result
∫f(x) dx = (x^2 + x + C) / (x^2 + 1) = x + C / (x^2 + 1)
Gallery of Quotient Rule Examples:






FAQ Section:
What is the quotient rule for integrals?
+The quotient rule for integrals is a method for integrating functions that can be expressed as the ratio of two functions.
How do I apply the quotient rule formula?
+To apply the quotient rule formula, substitute the derivative of h(x) and the integral of g(x) into the quotient rule formula.
What are some common mistakes to avoid when using the quotient rule?
+Common mistakes to avoid when using the quotient rule include failing to identify the functions g(x) and h(x), incorrectly evaluating the derivative of h(x), and failing to simplify the result.
We hope this article has helped you master the quotient rule for integrals in 5 easy steps. With practice and patience, you can become proficient in using this powerful technique to solve complex integration problems. Don't forget to try out the example problems and explore the gallery of quotient rule examples to further reinforce your understanding. Happy learning!