Quadratic Equations In Intercept Form Made Easy

Quick Links :

Understanding quadratic equations in intercept form is a fundamental concept in algebra, and it's essential to grasp it to solve various problems in mathematics and science. In this article, we'll delve into the world of quadratic equations in intercept form, exploring what they are, their benefits, and how to work with them.

What are Quadratic Equations in Intercept Form?

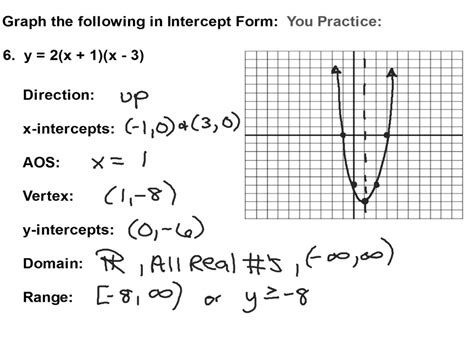
A quadratic equation in intercept form is a polynomial equation of degree two, where the x-intercepts are explicitly given. The general form of a quadratic equation in intercept form is:

y = a(x - p)(x - q)

where p and q are the x-intercepts, and a is a coefficient that determines the direction and width of the parabola.

Benefits of Quadratic Equations in Intercept Form

1. Easy to identify x-intercepts: The x-intercepts are explicitly given in the equation, making it easy to identify the points where the parabola intersects the x-axis.
2. Simple to graph: With the x-intercepts known, graphing the parabola becomes a straightforward process.
3. Efficient for problem-solving: Quadratic equations in intercept form are ideal for solving problems that involve finding the x-intercepts or the vertex of the parabola.

How to Work with Quadratic Equations in Intercept Form

To work with quadratic equations in intercept form, follow these steps:

1. Identify the x-intercepts: Look for the values of p and q in the equation.
2. Determine the coefficient a: Identify the value of a, which determines the direction and width of the parabola.
3. Graph the parabola: Use the x-intercepts and the coefficient a to graph the parabola.
4. Solve problems: Use the quadratic equation in intercept form to solve problems that involve finding the x-intercepts or the vertex of the parabola.

Example Problems

1. Find the x-intercepts of the quadratic equation y = 2(x - 3)(x + 2).
2. Graph the quadratic equation y = -3(x - 1)(x - 4).
3. Find the vertex of the quadratic equation y = 4(x - 2)(x + 1).

Real-World Applications of Quadratic Equations in Intercept Form

Quadratic equations in intercept form have numerous real-world applications, including:

1. Projectile motion: Quadratic equations in intercept form are used to model the trajectory of projectiles, such as the path of a thrown ball or the trajectory of a rocket.
2. Electrical engineering: Quadratic equations in intercept form are used to analyze and design electronic circuits.
3. Computer science: Quadratic equations in intercept form are used in computer graphics and game development to create realistic simulations.

Conclusion

Quadratic equations in intercept form are a powerful tool for solving problems in mathematics and science. By understanding the benefits and how to work with these equations, you'll be able to tackle a wide range of problems with ease. Whether you're a student, a teacher, or a professional, mastering quadratic equations in intercept form will open up new possibilities for you.

We hope this article has helped you understand quadratic equations in intercept form and their significance in mathematics and science. If you have any questions or comments, please feel free to share them below.