Conic Sections Explained: Understanding Polar Functions Easily

Quick Links :

Understanding conic sections and polar functions can be a daunting task, especially for students who are new to mathematics. However, with the right approach and resources, it can be made easier. In this article, we will delve into the world of conic sections and polar functions, exploring their importance, types, and applications.

What are Conic Sections?

Conic sections are a type of curve that can be obtained by intersecting a cone with a plane. They are called "conic" because they are derived from a cone. The shape of the curve depends on the angle of the plane and the cone. There are four main types of conic sections: circles, ellipses, parabolas, and hyperbolas.

Types of Conic Sections

Each type of conic section has its unique properties and applications.

• Circles: A circle is a set of points that are equidistant from a fixed point called the center. It is a special type of ellipse where the major and minor axes are equal.
• Ellipses: An ellipse is a set of points where the sum of the distances from two fixed points (called foci) is constant. It is used in astronomy to describe the orbits of planets and stars.
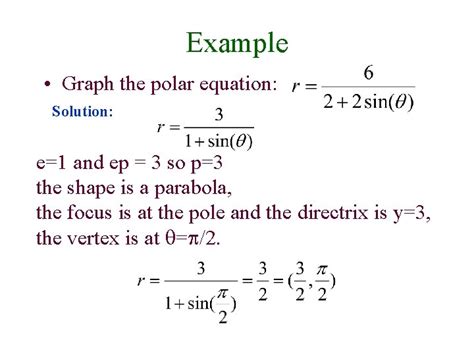
• Parabolas: A parabola is a set of points where the distance from a fixed point (called the focus) is equal to the distance from a fixed line (called the directrix). It is used in architecture to design arches and bridges.
• Hyperbolas: A hyperbola is a set of points where the difference between the distances from two fixed points (called foci) is constant. It is used in physics to describe the motion of objects at high speeds.

What are Polar Functions?

Polar functions are a way of describing curves using polar coordinates. In polar coordinates, a point is described by its distance from the origin (called the radius) and the angle from the positive x-axis (called the theta). Polar functions are useful for describing curves that have symmetry about the origin.

Types of Polar Functions

There are several types of polar functions, including:

• Circular Functions: These are functions that describe circles in polar coordinates. They are useful for describing circular motion and periodic phenomena.
• Spiral Functions: These are functions that describe spirals in polar coordinates. They are useful for describing growth and decay phenomena.
• Rose Curves: These are functions that describe rose-shaped curves in polar coordinates. They are useful for describing periodic phenomena with multiple frequencies.

Applications of Conic Sections and Polar Functions

Conic sections and polar functions have numerous applications in various fields, including:

• Astronomy: Conic sections are used to describe the orbits of planets and stars. Polar functions are used to describe the motion of celestial bodies in polar coordinates.
• Physics: Conic sections are used to describe the motion of objects under the influence of gravity. Polar functions are used to describe the motion of objects in polar coordinates.
• Engineering: Conic sections are used to design arches and bridges. Polar functions are used to design circular and spiral structures.
• Computer Science: Conic sections and polar functions are used in computer graphics to create smooth curves and surfaces.

In conclusion, conic sections and polar functions are powerful tools for describing curves and surfaces in mathematics. They have numerous applications in various fields, including astronomy, physics, engineering, and computer science. By understanding these concepts, we can gain insights into the natural world and develop new technologies and innovations.

We hope this article has provided you with a comprehensive understanding of conic sections and polar functions. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and comments below, and let's continue the conversation.