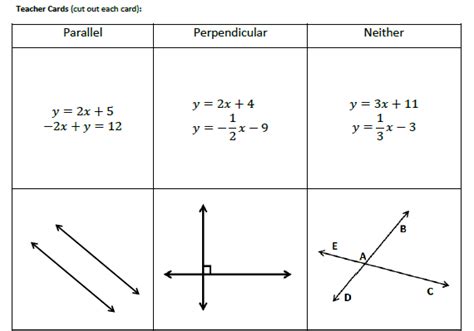
Understanding the relationships between lines is a fundamental concept in geometry and mathematics. The relationship between two lines can be described as parallel, perpendicular, or neither. In this article, we will delve into the world of line relationships and explore how to determine whether two lines are parallel, perpendicular, or neither.
Lines are the foundation of geometry, and their relationships are crucial in various mathematical and real-world applications. From designing buildings to calculating distances, understanding line relationships is essential. In this article, we will discuss the concepts of parallel, perpendicular, and neither lines, and provide a comprehensive guide on how to determine the relationship between two lines using a line relationship calculator.
What are Parallel Lines?
Parallel lines are two lines that lie in the same plane and never intersect, no matter how far they are extended. In other words, parallel lines have the same slope and are always at the same distance from each other. The concept of parallel lines is crucial in geometry, as it helps us understand the relationships between shapes and objects.

Properties of Parallel Lines
Parallel lines have several key properties:
- They have the same slope
- They never intersect
- They are always at the same distance from each other
- They have the same angle of inclination
What are Perpendicular Lines?
Perpendicular lines, on the other hand, are two lines that intersect at a right angle (90 degrees). In other words, perpendicular lines have slopes that are negative reciprocals of each other. The concept of perpendicular lines is essential in geometry, as it helps us understand the relationships between shapes and objects.

Properties of Perpendicular Lines
Perpendicular lines have several key properties:
- They intersect at a right angle (90 degrees)
- They have slopes that are negative reciprocals of each other
- They are always at a right angle to each other
What are Neither Parallel nor Perpendicular Lines?
Neither parallel nor perpendicular lines are two lines that do not have the same slope and do not intersect at a right angle. In other words, these lines have different slopes and are not perpendicular to each other.

Properties of Neither Parallel nor Perpendicular Lines
Neither parallel nor perpendicular lines have several key properties:
- They have different slopes
- They do not intersect at a right angle
- They are not parallel to each other
Line Relationship Calculator
Now that we have discussed the concepts of parallel, perpendicular, and neither lines, let's explore how to determine the relationship between two lines using a line relationship calculator.
A line relationship calculator is a tool that helps you determine the relationship between two lines based on their slopes. The calculator takes the slopes of the two lines as input and outputs the relationship between the lines.
Here's how to use a line relationship calculator:
- Enter the slopes of the two lines
- Click the "Calculate" button
- The calculator will output the relationship between the lines (parallel, perpendicular, or neither)
Example
Let's say we have two lines with slopes 2 and 3. We can use a line relationship calculator to determine the relationship between the lines.
Enter the slopes of the lines: 2 and 3 Click the "Calculate" button The calculator outputs: Neither parallel nor perpendicular
This means that the two lines are neither parallel nor perpendicular to each other.
Conclusion
In conclusion, understanding the relationships between lines is a fundamental concept in geometry and mathematics. The relationship between two lines can be described as parallel, perpendicular, or neither. By using a line relationship calculator, we can determine the relationship between two lines based on their slopes.
We hope this article has helped you understand the concepts of parallel, perpendicular, and neither lines, and how to use a line relationship calculator to determine the relationship between two lines.






What is the relationship between two lines with the same slope?
+Two lines with the same slope are parallel to each other.
What is the relationship between two lines that intersect at a right angle?
+Two lines that intersect at a right angle are perpendicular to each other.
How can I determine the relationship between two lines using a line relationship calculator?
+Enter the slopes of the two lines into the calculator, and it will output the relationship between the lines (parallel, perpendicular, or neither).