Normal Distribution Table Pdf: A Quick Reference Guide

Quick Links :

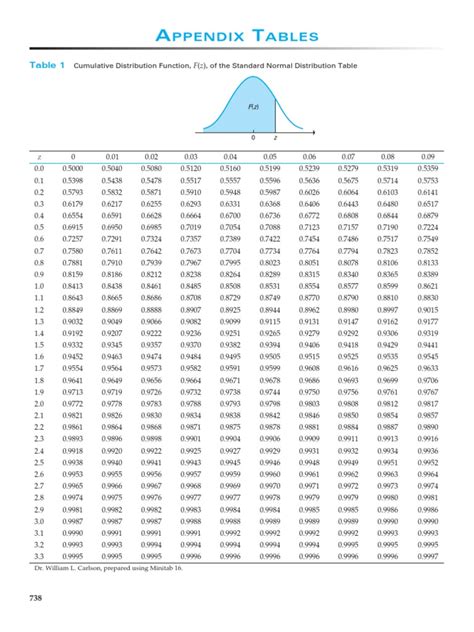
The normal distribution table, also known as the z-table, is a fundamental tool in statistics and data analysis. It provides a quick and easy way to determine the probability of a random variable falling within a certain range of values. In this article, we will explore the normal distribution table in more detail, including its history, how to use it, and provide a downloadable PDF version for your convenience.

What is the Normal Distribution Table?

The normal distribution table is a statistical table that lists the probabilities of a normal distribution. It is also known as the standard normal distribution table or the z-table. The table provides the area under the standard normal curve to the left of a given z-score. The z-score is a measure of how many standard deviations an observation is away from the mean.

History of the Normal Distribution Table

The normal distribution table has its roots in the 18th century when mathematician and astronomer Pierre-Simon Laplace first proposed the idea of a normal distribution. However, it wasn't until the early 20th century that the table was first developed by statistician and mathematician Karl Pearson. Since then, the table has undergone several revisions and improvements, with the most recent version being widely used today.

How to Use the Normal Distribution Table

Using the normal distribution table is relatively straightforward. Here are the steps:

1. Determine the z-score: Calculate the z-score of the value you are interested in. The z-score is calculated using the formula: z = (X - μ) / σ, where X is the value, μ is the mean, and σ is the standard deviation.
2. Look up the z-score: Find the z-score in the table. The table lists the z-scores in the first column.
3. Determine the probability: Read off the probability from the table. The table lists the probabilities in the second column.

For example, suppose we want to determine the probability of a value being less than 1.5 standard deviations above the mean. We would calculate the z-score as follows: z = (1.5 - 0) / 1 = 1.5. We would then look up the z-score in the table and find the corresponding probability.

Interpreting the Normal Distribution Table

The normal distribution table provides the area under the standard normal curve to the left of a given z-score. This means that the table gives us the probability of a value being less than or equal to a certain z-score.

For example, if we look up a z-score of 1.5 in the table, we find that the probability is 0.9332. This means that 93.32% of the values in a normal distribution will be less than or equal to 1.5 standard deviations above the mean.

Limitations of the Normal Distribution Table

While the normal distribution table is a powerful tool, it does have some limitations. One of the main limitations is that it assumes a normal distribution, which may not always be the case in real-world data. Additionally, the table is only applicable for standard normal distributions, which means that the data must have a mean of 0 and a standard deviation of 1.

Downloadable Normal Distribution Table PDF

For your convenience, we have provided a downloadable PDF version of the normal distribution table. You can download it here: [insert link to PDF]

Gallery of Normal Distribution Tables

FAQs

We hope this article has provided a comprehensive guide to the normal distribution table. Whether you are a student, researcher, or professional, understanding how to use the normal distribution table is an essential skill in statistics and data analysis.