Mastering The Laplace Transform Of Delta Function

Quick Links :

The Laplace transform is a powerful mathematical tool used to solve differential equations and integral equations. It has numerous applications in various fields, including engineering, physics, and mathematics. One of the fundamental concepts in Laplace transform is the delta function, also known as the Dirac delta function. In this article, we will explore the Laplace transform of the delta function, its properties, and applications.

Understanding the Delta Function

The delta function, denoted by δ(t), is a mathematical concept that represents an infinitely narrow and infinitely tall spike at the origin. It is defined as:

δ(t) = 0 for t ≠ 0 δ(t) = ∞ for t = 0

The delta function has several important properties, including:

• The delta function is an even function, meaning δ(-t) = δ(t)
• The delta function is a linear function, meaning δ(at) = (1/|a|)δ(t)
• The delta function has a unit area, meaning ∫∞ -∞ δ(t) dt = 1

Laplace Transform of the Delta Function

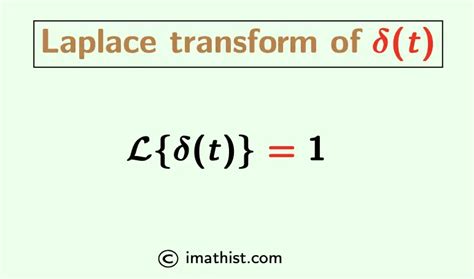
The Laplace transform of the delta function is given by:

ℒ[δ(t)] = ∫∞ 0 δ(t)e^{-st} dt

Using the definition of the delta function, we can simplify the integral to:

ℒ[δ(t)] = e^0 ℒ[δ(t)] = 1

Therefore, the Laplace transform of the delta function is equal to 1.

Properties of the Laplace Transform of the Delta Function

The Laplace transform of the delta function has several important properties, including:

• Linearity: ℒ[αδ(t)] = αℒ[δ(t)]
• Shifting: ℒ[δ(t-a)] = e^{-as}
• Scaling: ℒ[δ(at)] = (1/|a|)e^{-st}

Applications of the Laplace Transform of the Delta Function

The Laplace transform of the delta function has numerous applications in various fields, including:

• Signal processing: The Laplace transform of the delta function is used to analyze and design filters, such as low-pass, high-pass, and band-pass filters.
• Control systems: The Laplace transform of the delta function is used to analyze and design control systems, such as feedback control systems.
• Communication systems: The Laplace transform of the delta function is used to analyze and design communication systems, such as modulation and demodulation systems.

Example Problems

1. Find the Laplace transform of the following function:

f(t) = δ(t) + 2e^{-t}

Solution:

ℒ[f(t)] = ℒ[δ(t)] + 2ℒ[e^{-t}] ℒ[f(t)] = 1 + 2/(s+1)

2. Find the inverse Laplace transform of the following function:

F(s) = 1/(s+2)

Solution:

ℒ^{-1}[F(s)] = ℒ^{-1}[1/(s+2)] ℒ^{-1}[F(s)] = e^{-2t}

Conclusion

In this article, we have explored the Laplace transform of the delta function, its properties, and applications. The Laplace transform of the delta function is a fundamental concept in mathematics and engineering, and it has numerous applications in various fields. By mastering the Laplace transform of the delta function, engineers and mathematicians can analyze and design complex systems, such as control systems and communication systems.

We hope this article has provided you with a comprehensive understanding of the Laplace transform of the delta function. If you have any questions or need further clarification, please feel free to ask.

Gallery of Laplace Transform of Delta Function

FAQ Section