Integrating Absolute Value Functions Made Easy

Quick Links :

The world of mathematics can be fascinating, yet daunting, especially when it comes to complex concepts like absolute value functions. However, with a little creativity and the right approach, even the most challenging topics can become accessible and enjoyable. In this article, we will explore the concept of absolute value functions, their applications, and provide practical examples to make learning and integrating them a breeze.

What are Absolute Value Functions?

Absolute value functions are a type of mathematical function that takes the absolute value of a given input. In other words, they return the magnitude or distance of the input from zero, without considering its direction or sign. These functions are essential in various fields, such as physics, engineering, economics, and computer science, where they help model real-world phenomena, make predictions, and optimize systems.

Types of Absolute Value Functions

There are several types of absolute value functions, including:

• Simple absolute value functions: These are the most basic type, where the absolute value of a single variable is taken.
• Piecewise absolute value functions: These functions involve multiple absolute value expressions, each applied to a different part of the domain.
• Absolute value functions with parameters: These functions include additional parameters that can be adjusted to change the shape and behavior of the function.

Applications of Absolute Value Functions

Absolute value functions have numerous applications in various fields, including:

• Physics: Absolute value functions are used to model the motion of objects, calculate distances, and determine the magnitude of forces.
• Engineering: These functions are employed in the design of electronic circuits, signal processing, and control systems.
• Economics: Absolute value functions are used to model economic systems, calculate costs, and determine the optimal allocation of resources.
• Computer Science: These functions are used in algorithms for data analysis, machine learning, and computer vision.

Real-World Examples of Absolute Value Functions

• Calculating the distance between two points on a coordinate plane
• Determining the magnitude of a force or acceleration
• Modeling the motion of an object under the influence of gravity
• Calculating the cost of producing a product based on the absolute value of the demand

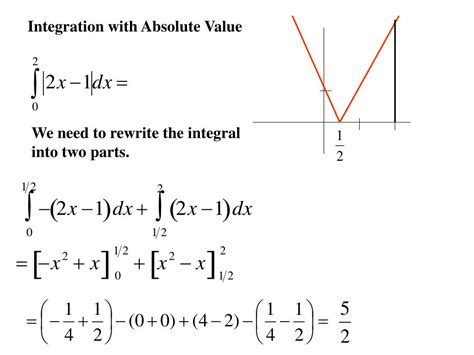
Integrating Absolute Value Functions

Integrating absolute value functions can be challenging, but with the right approach, it can become manageable. Here are some tips to help you integrate absolute value functions:

• Break down the function into smaller parts, if possible
• Use substitution or change of variables to simplify the function
• Apply the fundamental theorem of calculus, which states that differentiation and integration are inverse processes
• Use the chain rule and the product rule to differentiate and integrate composite functions

Step-by-Step Examples of Integrating Absolute Value Functions

• Integrate the function f(x) = |x| from x = -1 to x = 1
• Integrate the function f(x) = |x^2 - 4| from x = -2 to x = 2
• Integrate the function f(x) = |sin(x)| from x = 0 to x = π

Conclusion and Final Thoughts

In conclusion, absolute value functions are a fundamental concept in mathematics, with numerous applications in various fields. Integrating these functions can be challenging, but with the right approach, it can become manageable. By breaking down the function into smaller parts, using substitution or change of variables, applying the fundamental theorem of calculus, and using the chain rule and the product rule, you can master the art of integrating absolute value functions.

We hope this article has provided you with a comprehensive understanding of absolute value functions and their applications. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences with integrating absolute value functions in the comments below.