5 Ways To Master Absolute Value Inequalities

Quick Links :

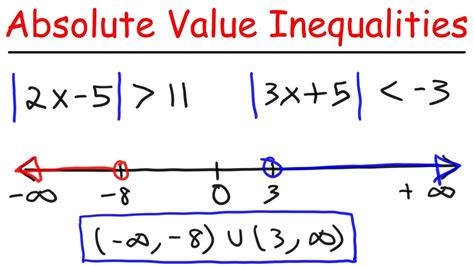
Mastering absolute value inequalities is a crucial aspect of mathematics, particularly in algebra and beyond. Absolute value inequalities can be challenging, but with the right strategies and techniques, you can become proficient in solving them. In this article, we will explore five ways to master absolute value inequalities, including understanding the concept, solving linear absolute value inequalities, graphing absolute value inequalities, using algebraic methods, and practicing with real-world applications.

Understanding the Concept of Absolute Value Inequalities

Before we dive into the methods of solving absolute value inequalities, it's essential to understand the concept. Absolute value inequalities involve finding the values of a variable that satisfy a given inequality involving absolute value. The absolute value of a number is its distance from zero on the number line, without considering direction. For example, the absolute value of -3 is 3, and the absolute value of 3 is also 3.

Definition of Absolute Value Inequalities

An absolute value inequality is an inequality that contains an absolute value expression. For example:

|2x + 1| > 5

This inequality states that the distance between 2x + 1 and zero is greater than 5.

Solving Linear Absolute Value Inequalities

Linear absolute value inequalities involve finding the values of a variable that satisfy a given inequality involving absolute value. The most common method for solving linear absolute value inequalities is to isolate the absolute value expression and then analyze the inequality.

Steps to Solve Linear Absolute Value Inequalities

1. Isolate the absolute value expression.
2. Set up two separate inequalities based on the definition of absolute value.
3. Solve each inequality separately.
4. Combine the solutions to find the final answer.

Graphing Absolute Value Inequalities

Graphing absolute value inequalities is an alternative method to solving linear absolute value inequalities. Graphing involves plotting the absolute value expression on a coordinate plane and analyzing the inequality.

Steps to Graph Absolute Value Inequalities

1. Plot the absolute value expression on a coordinate plane.
2. Draw a line or curve to represent the absolute value expression.
3. Analyze the inequality by determining the region that satisfies the inequality.
4. Write the final answer in interval notation.

Using Algebraic Methods to Solve Absolute Value Inequalities

Algebraic methods involve using algebraic techniques to solve absolute value inequalities. This method is particularly useful for solving more complex absolute value inequalities.

Steps to Use Algebraic Methods

1. Isolate the absolute value expression.
2. Use algebraic techniques, such as factoring or the quadratic formula, to solve the inequality.
3. Analyze the solutions to determine the final answer.

Practicing with Real-World Applications

Practicing with real-world applications is an excellent way to reinforce your understanding of absolute value inequalities. Real-world applications involve using absolute value inequalities to solve problems in physics, engineering, economics, and other fields.

Examples of Real-World Applications

1. Physics: Absolute value inequalities can be used to determine the distance between objects.
2. Engineering: Absolute value inequalities can be used to design electronic circuits.
3. Economics: Absolute value inequalities can be used to model economic systems.

In conclusion, mastering absolute value inequalities requires practice, patience, and persistence. By following the five methods outlined in this article, you can become proficient in solving absolute value inequalities and applying them to real-world applications. Remember to always analyze the inequality carefully, use the correct method, and check your solutions to ensure accuracy.